Multiplying on an Abacus

Continuing with different ways to multiply, here’s just the one

(18) The Abacus.  To start, you need to know how to write numbers on the Chinese abacus.  Numbers are written with the 1s column on the far right, then the 10s column, then the 100s column, etc.  and you only look at which beads are touching the center bar.  Beads on the top count as 5, while beads on the bottom count as 1.  Here’s the number 358:

Notice that you could get by with each rod only having 1 bead on the top and 4 on the bottom.  Some have that; it’s called a 1/4 abacus, and I believe it’s been popular in Japan for about 60 or 70 years.  It’s called a soroban.  The 2/5 abacus (which is what I have) is used in China, and it’s called a Saun-pan.  It just makes certain carrying easier, plus it’s what I could find.

On to adding!  Some of this is easy:  If you have a number like 358 and you want to add 100, you just move one of the 100 beads [third column from the right].

But what if it’s not that simple?  If you have a number like 358 and you want to add 18, you could do it bead by bead (Slow.   Boring.  Lots of regrouping.)  or you could just add 2 tens and then remove 2 ones.  That’s the more authentic way.

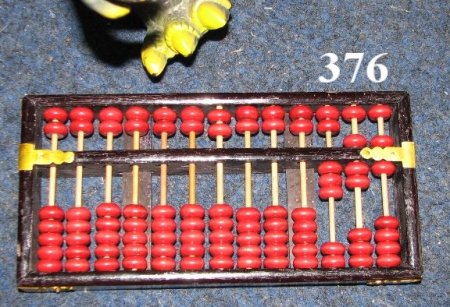
Likewise, if you had 358 and you wanted to add 4, you could add a five bead and subtract 1 unit, then regroup, or you could add 1 ten bead and subtract the five and a one bead.    [Is that even clear?  You can find java versions online to follow along, like this one although it uses two decimal places on the far right instead of starting with the 1s.]

On to multiplication!  For single digits, well, you pretty much just need to know all your basic multiplication facts.  No way around it.  But this process will work for anything beyond that.

Let’s find 87×625.  Pick one of the numbers (87) and put it on the far left-hand side of the abacus.  Since 87 is a 2 digit number, we want to leave 3 blank spaces that’s (2+1) on the far right, and we’ll put 625 after that (where by “after” I guess I mean “before”, to the left).  Just look at the picture and it should be clear.

(Pause.)  Before we get started on the process, it’s helpful to take a look at the big picture.  We’re going to put our answer on the far right, which is why we needed those blank spaces.  But our answer will be more than 3 digits long, so we’re going to need more space.  We’ll create the extra space as we move along.  We’ll first multiply 87 by the 5 of 625 then we’ll get rid of that 5, freeing up an extra column.  then we’ll multiply 87 by the 2 of 625, freeing up yet another column, and finally we’ll multiply the 87 by the 6.

Let’s get started.

We want to multiply 87 by the 5.  We’ll multiply the digit 8 and then the digit 7 [left to right].  Yes, the is backwards from how we’re multiplying 625 [right to left], but that just keeps it fun.   Whenever we multiply by the 8 of 87 we’ll put the answer two columns over, and whenever we multiply by the 7 of 87 we’ll put the answer three columns over.   [No matter how many digits that number is, when you multiply by the largest place-value you put the answer two columns over; the next place value goes three columns over; the next would go four columns over, etc.]

So to start, we know that 8×5 is 40.  Since we’re using the 8, we’ll put the answer two columns over from the 5.  We always use two for the left-most column.

Now we’ll move to 7×5.  This is 35, and we put the answer three columns over from the 5.

Since we’re done multiplying 87 by 5, we can remove the 5.

Notice the number on the far right, 435, is just 87×5.

We’ll do the same thing, multiplying 87 by 2.  First we find 8×2 and put that answer two columns over from the 2.  [I’m going to do that by adding 20 instead of 16, and then subtracting 4.]

Then we’ll multiply 7×2 and put that answer three columns over from the 2.  [Here I added 15 instead of 14, and then I subtracted 1.]

We’re done multiplying by 2, so we can remove it.  Except I forgot to take a picture with it removed.  If you ignore that 2 of 62 (formerly 625), you can see that the number on the far right is now 2175.  Sure enough, this is 87×25.

Nearly done, we’ll multiply the 8 of 87 by 6 and put the answer two columns over from 6.  Notice that this 6 originally stood for 600 (in 625), but we don’t need to keep track of which place value the 6 originally had.  By always putting the single-digit products two or three (or four for longer numbers) over, the place value takes care of itself.

And finally, we multiply the 7 of 87 by the 6 and put the answer three columns over from 6.

And remove the 6 because we’re done multiplying by it.  Here’s the final answer:

Yes, it’s 54,375.  And a quick check shows that   I made a mistake so I had to redo all of the photos I took, and then I made another mistake and had to retake more photos, but on the third time It’s right!  And despite how long it takes to explain, it really isn’t too bad to do by hand even if you’re learning it for the first time.